3 Of 16 Is What Percent
Webtuts
May 09, 2025 · 4 min read
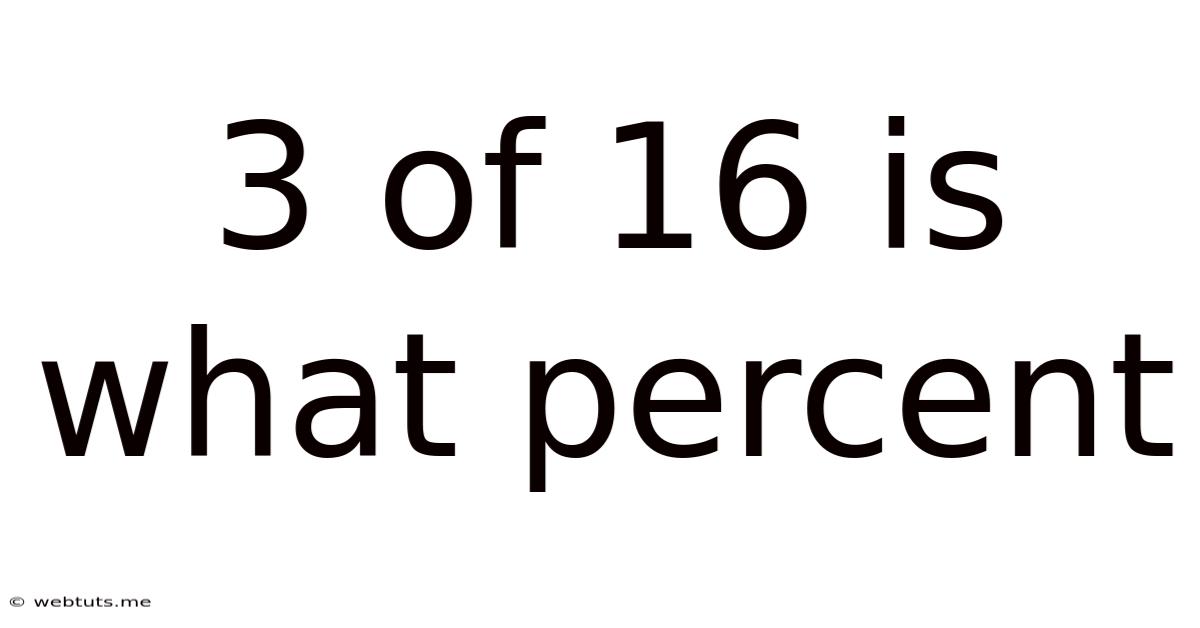
Table of Contents
3 of 16 is What Percent? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous areas of life, from calculating discounts and taxes to analyzing data and understanding statistics. This comprehensive guide will not only answer the question "3 of 16 is what percent?" but also provide you with a thorough understanding of percentage calculations, equipping you with the tools to solve similar problems independently.
Understanding Percentages: The Basics
A percentage represents a fraction of 100. The term "percent" literally means "out of 100." When we say "x percent," we mean x parts out of 100 equal parts. For instance, 50% represents 50 out of 100, which simplifies to 1/2 or 0.5.
The key to understanding percentages is to grasp the relationship between fractions, decimals, and percentages. They are all different ways of expressing the same proportion.
- Fraction: Represents a part of a whole, expressed as a ratio (e.g., 3/16).
- Decimal: Represents a part of a whole, expressed as a number less than 1 (e.g., 0.1875).
- Percentage: Represents a part of a whole, expressed as a number out of 100 (e.g., 18.75%).
These three forms are interchangeable, and mastering the conversion between them is crucial for proficient percentage calculations.
Calculating "3 of 16 is What Percent?"
Let's tackle the central question: What percentage is 3 out of 16? We can solve this using several methods:
Method 1: Using Proportions
This method leverages the fundamental concept of percentages as a ratio out of 100. We set up a proportion:
3/16 = x/100
Where 'x' represents the percentage we want to find. To solve for x, we cross-multiply:
16x = 300
x = 300/16
x = 18.75
Therefore, 3 of 16 is 18.75%.
Method 2: Converting to Decimal First
This method involves converting the fraction 3/16 to a decimal and then multiplying by 100 to express it as a percentage.
-
Convert the fraction to a decimal: Divide 3 by 16: 3 ÷ 16 = 0.1875
-
Multiply by 100 to express as a percentage: 0.1875 x 100 = 18.75%
Therefore, 3 of 16 is 18.75%.
Method 3: Using a Calculator
Most calculators have a percentage function. Simply enter 3 ÷ 16 and then multiply the result by 100. This gives you the same answer: 18.75%.
Expanding Your Percentage Calculation Skills
Understanding the basic calculation is just the beginning. Let's explore more complex scenarios and techniques:
Calculating Percentage Increase or Decrease
Percentage changes are crucial in various contexts, such as comparing sales figures, analyzing population growth, or tracking investment returns.
Formula for Percentage Increase:
[(New Value - Old Value) / Old Value] x 100
Formula for Percentage Decrease:
[(Old Value - New Value) / Old Value] x 100
Example: If sales increased from 100 units to 120 units, the percentage increase is:
[(120 - 100) / 100] x 100 = 20%
Finding the Original Value after a Percentage Change
This involves working backward from a percentage change to determine the initial value.
Formula (for percentage increase):
Original Value = New Value / (1 + Percentage Increase/100)
Formula (for percentage decrease):
Original Value = New Value / (1 - Percentage Decrease/100)
Example: If a price increased by 10% to $110, the original price was:
Original Value = $110 / (1 + 10/100) = $100
Calculating Percentage of a Percentage
This involves finding a percentage of a given percentage. For instance, finding 20% of 50%.
Method: Convert percentages to decimals and multiply:
(0.20) x (0.50) = 0.10 or 10%
Practical Applications of Percentage Calculations
Percentages are used extensively in various fields:
- Finance: Calculating interest rates, loan repayments, and investment returns.
- Retail: Determining discounts, sales tax, and profit margins.
- Statistics: Analyzing data, interpreting survey results, and presenting findings.
- Science: Expressing concentrations, calculating experimental yields, and representing data.
- Everyday Life: Calculating tips, splitting bills, and understanding discounts.
Common Mistakes to Avoid
- Incorrect order of operations: Remember the order of operations (PEMDAS/BODMAS) when performing calculations involving multiple operations.
- Confusing percentage increase/decrease: Ensure you are using the correct formula based on whether it's an increase or decrease.
- Rounding errors: Rounding off intermediate results can lead to inaccuracies in the final answer. Try to avoid rounding until the very end of your calculations.
- Not converting to decimals properly: Remember to convert percentages to decimals (divide by 100) before performing calculations.
Conclusion: Mastering the Power of Percentages
This comprehensive guide has not only answered the question "3 of 16 is what percent?" (18.75%) but has also equipped you with the knowledge and tools to tackle a wide range of percentage calculations. By understanding the underlying principles, mastering the different methods, and avoiding common pitfalls, you can confidently use percentages in various personal and professional contexts. Remember that practice is key; the more you work with percentages, the more comfortable and proficient you will become. So grab a calculator, some practice problems, and start honing your percentage calculation skills! You'll be surprised how frequently this seemingly simple skill comes in handy.
Latest Posts
Latest Posts
-
How Many More Days Until October 24th
May 09, 2025
-
21 Inches Is How Many Feet
May 09, 2025
-
How Many Tbsp Is 8 Tsp
May 09, 2025
-
How Much More Days Until Spring
May 09, 2025
-
How Many Inches In 1 Cubic Feet
May 09, 2025
Related Post
Thank you for visiting our website which covers about 3 Of 16 Is What Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.