How To Find Cubic Feet Of A Circle
Webtuts
Apr 07, 2025 · 5 min read
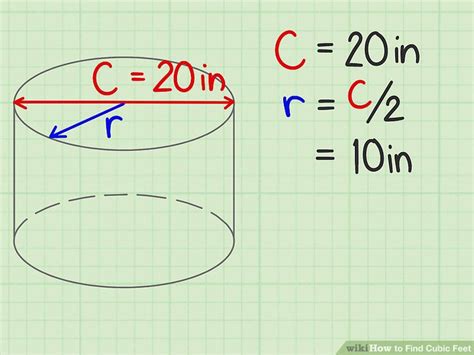
Table of Contents
How to Find Cubic Feet of a Circle: A Comprehensive Guide
Finding the cubic feet of a circle might seem confusing at first. The key is understanding that a circle itself is a two-dimensional shape; it only has area, not volume. To find cubic feet, we need a three-dimensional object. This means we're actually calculating the volume of a cylinder (a circle extended into a height) or a sphere (a three-dimensional circle). This guide will walk you through both calculations.
Keywords: cubic feet, circle volume, cylinder volume, sphere volume, volume calculation, cubic feet calculation, three-dimensional shapes, geometric volume, area, radius, diameter, height, pi, formula
Understanding the Basics: Area vs. Volume
Before diving into the calculations, let's clarify the difference between area and volume:
- Area: Measures the space inside a two-dimensional shape like a circle or square. It's measured in square units (e.g., square feet, square meters).
- Volume: Measures the space inside a three-dimensional shape like a cylinder, sphere, or cube. It's measured in cubic units (e.g., cubic feet, cubic meters).
Calculating the Volume of a Cylinder (Circular Prism)
A cylinder is essentially a circle extended to a specific height. To find its volume in cubic feet, we use the following formula:
Volume (Cylinder) = π * r² * h
Where:
- π (pi): A mathematical constant, approximately 3.14159.
- r: The radius of the circular base (half of the diameter).
- h: The height of the cylinder.
Step-by-Step Calculation:
-
Measure the radius (r): Use a ruler or measuring tape to find the distance from the center of the circle to its edge. Remember to convert your measurement to feet if it's in inches or any other unit.
-
Measure the height (h): Measure the vertical distance from the top to the bottom of the cylinder in feet.
-
Square the radius (r²): Multiply the radius by itself (r * r).
-
Multiply by π: Multiply the squared radius by π (approximately 3.14159).
-
Multiply by the height (h): Multiply the result from step 4 by the height of the cylinder.
-
The result is the volume in cubic feet: This final number represents the volume of your cylinder in cubic feet.
Example:
Let's say we have a cylinder with a radius of 2 feet and a height of 5 feet.
- r = 2 feet
- h = 5 feet
- r² = 2 * 2 = 4 square feet
- π * r² = 3.14159 * 4 ≈ 12.566 square feet
- Volume = 12.566 * 5 ≈ 62.83 cubic feet
Therefore, the cylinder has a volume of approximately 62.83 cubic feet.
Calculating the Volume of a Sphere
A sphere is a perfectly round three-dimensional object. Its volume is calculated using a slightly different formula:
Volume (Sphere) = (4/3) * π * r³
Where:
- π (pi): Again, approximately 3.14159.
- r: The radius of the sphere.
Step-by-Step Calculation:
-
Measure the radius (r): Find the distance from the center of the sphere to its edge. Convert the measurement to feet if necessary.
-
Cube the radius (r³): Multiply the radius by itself three times (r * r * r).
-
Multiply by π: Multiply the cubed radius by π (approximately 3.14159).
-
Multiply by (4/3): Multiply the result from step 3 by (4/3) or 1.3333.
-
The result is the volume in cubic feet: This final number is the volume of your sphere in cubic feet.
Example:
Let's say we have a sphere with a radius of 1 foot.
- r = 1 foot
- r³ = 1 * 1 * 1 = 1 cubic foot
- π * r³ = 3.14159 * 1 ≈ 3.142 cubic feet
- Volume = 3.142 * (4/3) ≈ 4.189 cubic feet
Therefore, the sphere has a volume of approximately 4.189 cubic feet.
Practical Applications: When to Use These Calculations
Understanding how to calculate the volume of cylinders and spheres has numerous practical applications:
-
Storage and Shipping: Determining the volume of cylindrical or spherical containers is crucial for calculating storage space needed in warehouses or for shipping goods efficiently.
-
Construction and Engineering: Calculating the volume of cylindrical pillars, spherical tanks, or other components is vital in construction and engineering projects.
-
Agriculture: Determining the volume of silos (cylindrical) or water tanks (spherical) is important for efficient resource management on farms.
-
Manufacturing: Calculating volumes of various cylindrical or spherical components during the manufacturing process is essential.
-
Science and Research: Calculating the volume of liquids or gases in cylindrical or spherical containers is crucial for various experiments and research purposes.
Advanced Considerations: Irregular Shapes and Approximations
The formulas above work perfectly for perfectly cylindrical or spherical objects. However, real-world objects might have slight irregularities. In such cases, you might need to make approximations. For example, you might need to break down an irregular shape into several smaller, simpler shapes (cylinders or spheres), calculate their volumes individually and then add them together to get an estimate of the total volume.
Troubleshooting and Common Mistakes
-
Units: Ensure all measurements are in feet before performing the calculations to get the final volume in cubic feet.
-
Radius vs. Diameter: Remember that the radius is half the diameter. Don't use the diameter directly in the formulas.
-
π Approximation: Using a more precise value of π (e.g., 3.14159265359) will give you a more accurate result, but for most practical purposes, 3.14159 is sufficient.
-
Calculator Use: Use a calculator for accurate calculations, especially when dealing with decimals and exponents.
Conclusion
Calculating the cubic feet of a circle (actually the volume of a cylinder or sphere) is a fundamental concept in geometry with wide-ranging practical applications. By understanding the formulas and following the steps outlined in this guide, you can accurately determine the volume of various three-dimensional shapes encountered in daily life, whether in home improvement, industrial settings, or scientific research. Remember to pay close attention to units, utilize the correct formula, and utilize a calculator for precise calculations. Mastering these calculations will enhance your problem-solving skills and give you valuable insights into the world of measurement and geometry.
Latest Posts
Latest Posts
-
What Is 9 Hours Ago From Now
Apr 08, 2025
-
How Long Ago Was 10 Weeks Ago
Apr 08, 2025
-
8 Weeks And 2 Days Ago From Today
Apr 08, 2025
-
How Many Pounds Is 36 Kg
Apr 08, 2025
-
What Is 25 Days From Now
Apr 08, 2025
Related Post
Thank you for visiting our website which covers about How To Find Cubic Feet Of A Circle . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.