Is 1 2 Greater Than 3 5
Webtuts
May 12, 2025 · 5 min read
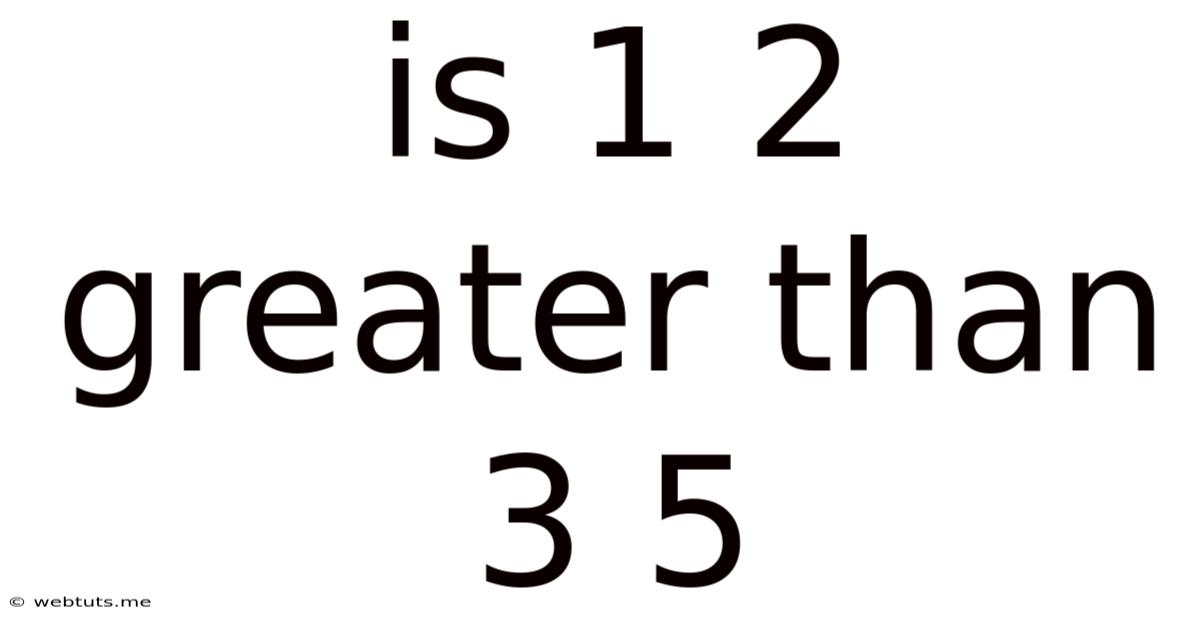
Table of Contents
Is 1/2 Greater Than 3/5? A Deep Dive into Fraction Comparison
The seemingly simple question, "Is 1/2 greater than 3/5?", opens a door to a broader understanding of fractions, their representation, and various methods for comparing them. While the answer might appear immediately obvious to some, a thorough exploration of the topic reveals valuable insights applicable to a range of mathematical concepts. This article will not only answer the question definitively but also equip you with the skills to compare any two fractions confidently.
Understanding Fractions: Building Blocks of Comparison
Before diving into the comparison, let's solidify our understanding of fractions. A fraction represents a part of a whole. It consists of two key components:
- Numerator: The top number indicates how many parts we have.
- Denominator: The bottom number indicates the total number of equal parts the whole is divided into.
For instance, in the fraction 1/2, the numerator (1) signifies that we possess one part, and the denominator (2) shows that the whole is divided into two equal parts. Similarly, 3/5 indicates that we have three parts out of a total of five equal parts.
Method 1: Finding a Common Denominator
This is perhaps the most common and intuitive method for comparing fractions. The principle lies in converting both fractions to equivalent fractions with the same denominator. This allows for a direct comparison of the numerators.
To find a common denominator for 1/2 and 3/5, we need to find the least common multiple (LCM) of 2 and 5. The LCM of 2 and 5 is 10.
Now, let's convert each fraction:
- 1/2: To get a denominator of 10, we multiply both the numerator and the denominator by 5: (1 * 5) / (2 * 5) = 5/10
- 3/5: To get a denominator of 10, we multiply both the numerator and the denominator by 2: (3 * 2) / (5 * 2) = 6/10
Now the comparison is straightforward: 5/10 is less than 6/10. Therefore, 1/2 is less than 3/5.
Method 2: Converting to Decimals
Another effective approach involves converting both fractions into their decimal equivalents. This method is particularly useful when dealing with more complex fractions or when a quick comparison is needed.
- 1/2: Dividing 1 by 2 gives us 0.5
- 3/5: Dividing 3 by 5 gives us 0.6
Comparing the decimal values, we see that 0.5 is less than 0.6. Hence, 1/2 is less than 3/5.
Method 3: Visual Representation
While less suitable for complex fractions, visual representation offers a strong intuitive understanding of fraction comparison. Imagine two identical circles.
- Representing 1/2: Divide one circle into two equal halves and shade one half.
- Representing 3/5: Divide the other circle into five equal parts and shade three parts.
A visual inspection reveals that the shaded area in the circle representing 3/5 is larger than the shaded area in the circle representing 1/2. Therefore, 1/2 is less than 3/5.
Method 4: Cross-Multiplication
This algebraic method provides a concise and efficient way to compare two fractions. Cross-multiply the numerators and denominators as follows:
- Multiply the numerator of the first fraction by the denominator of the second fraction: 1 * 5 = 5
- Multiply the numerator of the second fraction by the denominator of the first fraction: 3 * 2 = 6
Compare the results: 5 < 6. Since the result of the first multiplication is less than the result of the second multiplication, the first fraction (1/2) is less than the second fraction (3/5). Therefore, 1/2 is less than 3/5.
Expanding the Understanding: Beyond Simple Comparisons
The methods outlined above are not just limited to comparing simple fractions like 1/2 and 3/5. They can be applied to more complex fractions, including those with larger numerators and denominators, mixed numbers (whole numbers combined with fractions), and even improper fractions (where the numerator is larger than the denominator).
For instance, consider comparing 7/12 and 5/8. Using the common denominator method, the LCM of 12 and 8 is 24. Converting the fractions:
- 7/12 = (7 * 2) / (12 * 2) = 14/24
- 5/8 = (5 * 3) / (8 * 3) = 15/24
Clearly, 14/24 < 15/24, so 7/12 < 5/8.
Real-World Applications: Where Fraction Comparison Matters
Understanding fraction comparison is crucial in various real-world scenarios:
- Cooking and Baking: Adjusting recipes often requires comparing fractions to ensure accurate ingredient proportions.
- Construction and Engineering: Precise measurements and calculations necessitate accurate fraction comparisons.
- Finance and Budgeting: Managing finances effectively involves comparing fractions representing portions of budgets and expenses.
- Data Analysis: Interpreting data often involves comparing fractions representing proportions or percentages.
Mastering Fraction Comparison: Practice and Resources
The key to mastering fraction comparison is consistent practice. Start with simpler fractions and gradually work your way up to more complex ones. There are numerous online resources, including interactive exercises and quizzes, that can help you hone your skills.
Conclusion: A Simple Question, Profound Insights
The initial question, "Is 1/2 greater than 3/5?", might seem trivial. However, exploring the different methods for comparing fractions has revealed a wealth of knowledge about fractions, their representation, and their practical applications. Whether using common denominators, decimal conversion, visual aids, or cross-multiplication, the ability to confidently compare fractions is a fundamental mathematical skill with far-reaching implications in various fields. Mastering this skill will undoubtedly enhance your mathematical proficiency and problem-solving abilities. Remember to practice regularly and utilize available resources to build your confidence and understanding. The more you practice, the easier and more intuitive fraction comparison will become.
Latest Posts
Latest Posts
-
How Many Days Till Aug 20
May 14, 2025
-
How Many More Days Till April 7
May 14, 2025
-
How Many Days To March 10
May 14, 2025
-
2 3 Cup Convert To Tablespoons
May 14, 2025
-
How Many Ounces In A 5 Pound Bag Of Flour
May 14, 2025
Related Post
Thank you for visiting our website which covers about Is 1 2 Greater Than 3 5 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.