What Is The Decimal Of 1/10
Webtuts
May 09, 2025 · 5 min read
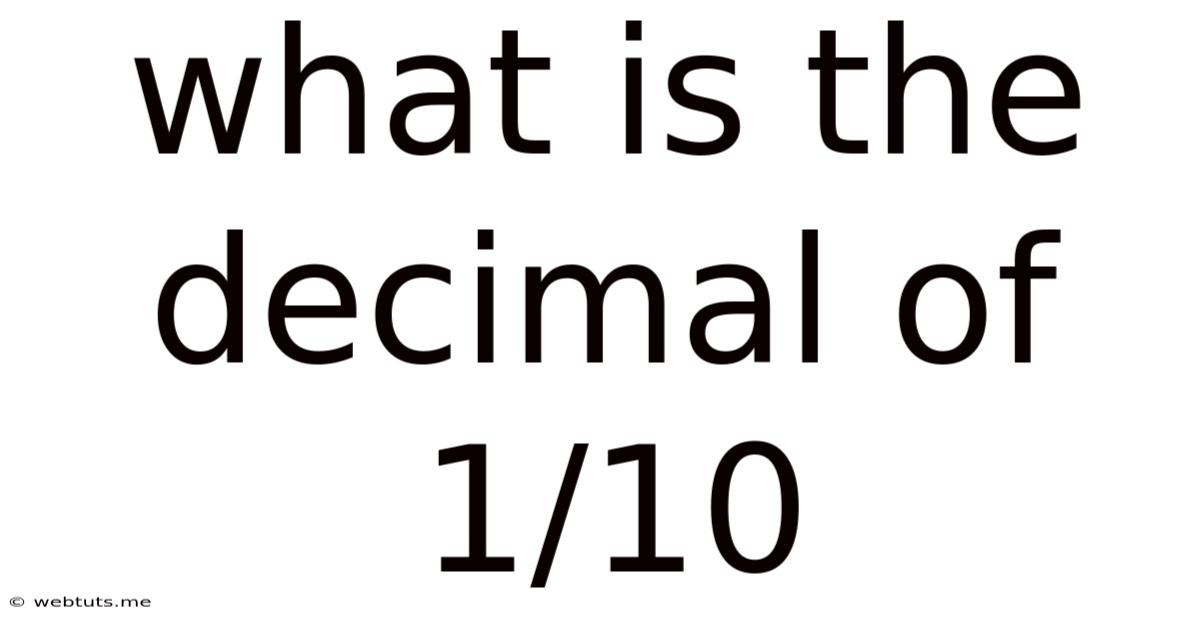
Table of Contents
What is the Decimal of 1/10? A Deep Dive into Decimal Conversion and its Applications
The seemingly simple question, "What is the decimal of 1/10?", opens the door to a fascinating exploration of decimal representation, fraction conversion, and the practical applications of these mathematical concepts in various fields. While the answer itself is straightforward (0.1), understanding the underlying principles and broader implications provides a rich learning experience. This article delves into the intricacies of decimal conversion, focusing on 1/10 as a foundational example, and explores its relevance in diverse contexts.
Understanding Fractions and Decimals
Before diving into the specific conversion of 1/10, let's establish a clear understanding of fractions and decimals.
Fractions represent parts of a whole. They consist of a numerator (the top number) and a denominator (the bottom number), separated by a horizontal line. The numerator indicates the number of parts we have, while the denominator indicates the total number of equal parts the whole is divided into. For instance, in the fraction 1/10, 1 is the numerator and 10 is the denominator. This fraction represents one part out of ten equal parts.
Decimals provide another way to represent parts of a whole. They use a base-ten system, where each digit to the right of the decimal point represents a decreasing power of ten. The first digit after the decimal point represents tenths (1/10), the second represents hundredths (1/100), the third represents thousandths (1/1000), and so on.
Converting 1/10 to a Decimal
Converting a fraction to a decimal involves dividing the numerator by the denominator. In the case of 1/10, we simply divide 1 by 10:
1 ÷ 10 = 0.1
Therefore, the decimal equivalent of 1/10 is 0.1. This is a fundamental concept in mathematics and forms the basis for understanding more complex decimal conversions.
The Significance of the Decimal Point
The decimal point is crucial in distinguishing between whole numbers and fractional parts. In 0.1, the digit '1' signifies one-tenth, a value less than one. The placement of the decimal point significantly affects the value of the number. For instance, 1.0 represents one whole unit, while 0.1 represents one-tenth of a unit. Understanding this distinction is critical for accurate calculations and problem-solving.
Expanding on the Concept: Other Decimal Conversions
While 1/10 provides a simple example, understanding the conversion process is crucial for tackling more complex fractions. Let's look at some examples:
- 1/2: 1 ÷ 2 = 0.5 (One-half is equivalent to five-tenths)
- 1/4: 1 ÷ 4 = 0.25 (One-quarter is equivalent to twenty-five hundredths)
- 1/5: 1 ÷ 5 = 0.2 (One-fifth is equivalent to two-tenths)
- 3/4: 3 ÷ 4 = 0.75 (Three-quarters is equivalent to seventy-five hundredths)
- 1/8: 1 ÷ 8 = 0.125 (One-eighth is equivalent to one hundred twenty-five thousandths)
These examples demonstrate how different fractions translate into their decimal equivalents. The process always involves dividing the numerator by the denominator. Sometimes, the division results in a terminating decimal (like the examples above), while other times, it results in a repeating decimal (e.g., 1/3 = 0.333...).
Practical Applications of Decimal Representation
The ability to convert fractions to decimals and vice versa is crucial in numerous real-world applications:
1. Finance and Accounting:
- Calculating percentages: Percentages are essentially fractions with a denominator of 100. Converting them to decimals simplifies calculations, such as finding discounts or interest rates. For example, 10% is equivalent to 0.1, making calculations easier.
- Working with monetary values: Decimals are essential for representing monetary amounts, as they accurately express cents or smaller units of currency. For instance, $0.10 represents ten cents.
- Analyzing financial statements: Financial statements often contain data expressed as fractions or percentages, which are readily converted to decimals for analysis and comparison.
2. Measurement and Science:
- Scientific measurements: Many scientific measurements are expressed in decimal form, ensuring precision and accuracy. For example, recording length, mass, or volume often involves decimals.
- Engineering and design: Engineers and designers utilize decimals extensively in calculations for construction, manufacturing, and other projects, guaranteeing accuracy and precision.
- Metric system: The metric system, widely used globally, relies heavily on decimal representation, simplifying conversions between units.
3. Everyday Life:
- Shopping and budgeting: Decimals are fundamental for understanding prices, calculating discounts, and managing personal finances.
- Cooking and baking: Recipes often involve fractional measurements that are easily converted to decimals for precision in cooking and baking.
- Data analysis: In various fields, from statistics to market research, data is often represented and analyzed using decimals.
Advanced Concepts and Further Exploration
Beyond the basic conversion of 1/10, several advanced concepts build upon this foundation:
- Repeating Decimals: Some fractions, like 1/3, result in repeating decimals (0.333...). Understanding these patterns requires exploring the concept of recurring decimals and their representation.
- Irrational Numbers: Numbers like π (pi) and √2 (the square root of 2) are irrational numbers, meaning they cannot be expressed as a fraction or as a terminating or repeating decimal. Understanding the nature of irrational numbers broadens mathematical knowledge.
- Binary and Other Number Systems: While our focus has been on the decimal (base-10) system, other number systems like the binary (base-2) system used in computers are also important. Comparing and contrasting different number systems provides a broader understanding of mathematical representation.
Conclusion
The seemingly simple question, "What is the decimal of 1/10?", serves as a springboard for a much deeper exploration of decimal representation, fraction conversion, and their wide-ranging applications. From basic financial calculations to advanced scientific measurements, the ability to comfortably work with decimals is a fundamental skill with significant real-world relevance. Understanding the principles discussed in this article provides a strong foundation for more advanced mathematical concepts and problem-solving across various disciplines. The simplicity of 1/10 = 0.1 belies the vast mathematical landscape it unlocks.
Latest Posts
Latest Posts
-
How Many Miles Is 400 Acres
May 09, 2025
-
How Many Cups Are In 20 Pints
May 09, 2025
-
How Many Pints In 1 Litre
May 09, 2025
-
30 Days From March 21 2024
May 09, 2025
-
How Many Pounds Is 50 Quarts
May 09, 2025
Related Post
Thank you for visiting our website which covers about What Is The Decimal Of 1/10 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.