What Is The Percent Of 4/7
Webtuts
May 09, 2025 · 5 min read
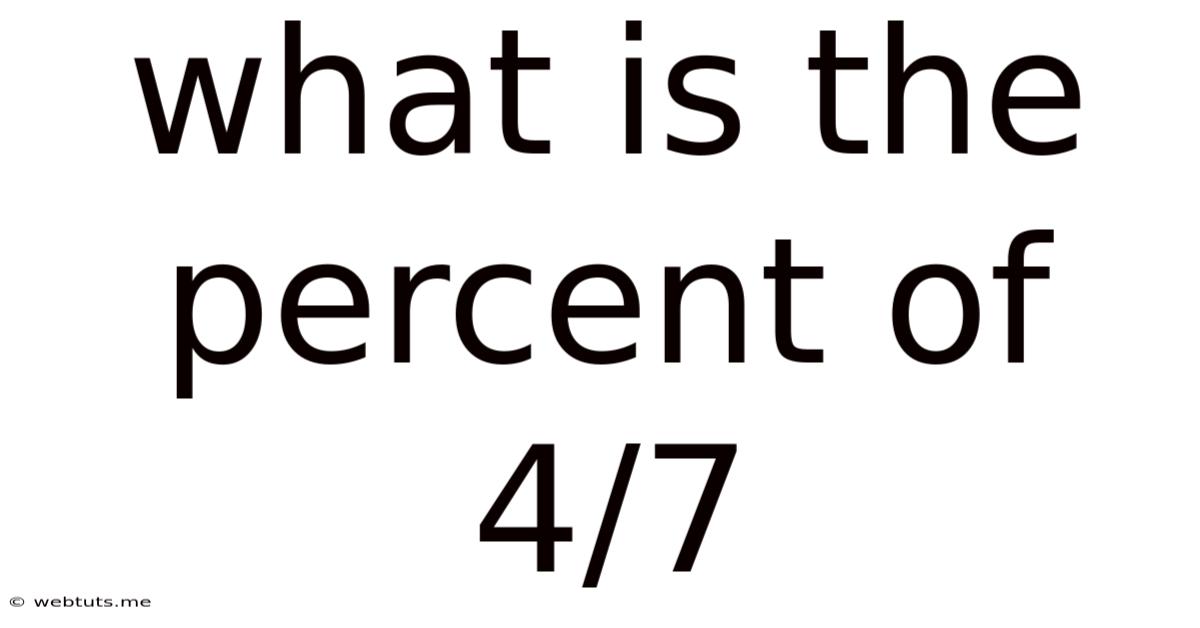
Table of Contents
What is the Percent of 4/7? A Comprehensive Guide to Fraction-to-Percentage Conversion
Understanding how to convert fractions into percentages is a fundamental skill in mathematics with broad applications in various fields. This comprehensive guide delves into the process of converting the fraction 4/7 into a percentage, explaining the steps involved and providing valuable insights into the underlying concepts. We'll explore different methods, discuss the significance of accuracy, and explore practical applications of this conversion.
Understanding Fractions and Percentages
Before diving into the conversion, let's refresh our understanding of fractions and percentages.
Fractions: A fraction represents a part of a whole. It consists of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator indicates the number of parts we have, while the denominator indicates the total number of parts in the whole. In our case, 4/7 means we have 4 parts out of a total of 7 parts.
Percentages: A percentage is a way of expressing a number as a fraction of 100. The symbol "%" represents "percent" or "per hundred." For example, 50% means 50 out of 100, or 50/100, which simplifies to 1/2.
Method 1: Direct Conversion Using Division
The most straightforward method to convert a fraction to a percentage is through division. Here's how to convert 4/7 to a percentage:
-
Divide the numerator by the denominator: Divide 4 by 7. This gives you 0.57142857... (the decimal continues infinitely).
-
Multiply the result by 100: Multiply the decimal obtained in step 1 by 100. This shifts the decimal point two places to the right. 0.57142857... x 100 = 57.142857...
-
Round to the desired level of accuracy: Since the decimal representation is non-terminating, we need to round the result. Commonly, percentages are rounded to two decimal places. Therefore, 4/7 is approximately 57.14%.
Method 2: Using Equivalent Fractions
Another approach involves creating an equivalent fraction with a denominator of 100. While not always feasible, this method offers a clearer conceptual understanding.
-
Find the multiplier: We need to determine what number we can multiply 7 by to get 100 (or a close approximation). This is not a whole number, but we can find an approximation. 100 / 7 ≈ 14.2857.
-
Multiply both numerator and denominator: Multiply both the numerator and the denominator of 4/7 by the approximate multiplier we found in step 1. This maintains the proportion of the fraction:
(4 x 14.2857) / (7 x 14.2857) ≈ 57.1428 / 100
-
Express as a percentage: Since the denominator is now approximately 100, the numerator directly represents the percentage. Therefore, 4/7 is approximately 57.14%. This method highlights the core concept of percentages as parts per hundred.
Understanding the Significance of Accuracy
The accuracy of the percentage depends on how many decimal places you retain during the calculations. Using a calculator, you'll get a more precise answer, but for practical purposes, rounding to two decimal places (57.14%) is usually sufficient. In applications requiring extreme accuracy, such as scientific calculations or financial modeling, more decimal places might be necessary.
Practical Applications of Fraction-to-Percentage Conversion
Converting fractions to percentages is crucial in various real-world scenarios:
-
Calculating Grades: If you answered 4 out of 7 questions correctly on a test, your score would be 57.14%.
-
Financial Calculations: Understanding percentages is vital in calculating interest rates, discounts, profit margins, and tax rates.
-
Data Analysis: Converting fractions to percentages is essential in representing data in charts and graphs, making it easier to visualize and interpret. For example, if 4 out of 7 people surveyed prefer a certain product, you can represent this as 57.14% preference.
-
Everyday Life: Percentages are used extensively in expressing discounts ("20% off"), sales tax, tips, and many other aspects of daily life.
Advanced Concepts and Related Calculations
While the conversion of 4/7 to a percentage is relatively straightforward, several advanced concepts build upon this fundamental skill:
-
Percentage Increase/Decrease: Calculating the percentage change between two values involves finding the difference, dividing by the original value, and multiplying by 100.
-
Compound Interest: Understanding compound interest involves repeated percentage calculations over time, demonstrating the power of exponential growth.
-
Statistical Analysis: Percentages are used extensively in statistical analysis to represent proportions, probabilities, and confidence intervals.
-
Ratio and Proportion: Fractions and percentages are intrinsically linked to the concept of ratios and proportions, which are crucial for solving many types of mathematical problems.
Improving Your Calculation Skills
To improve your ability to perform fraction-to-percentage conversions and other related calculations, consider the following:
-
Practice regularly: The more you practice, the more confident and proficient you will become. Work through various examples and try different methods.
-
Use a calculator effectively: Calculators are valuable tools, but it's crucial to understand the underlying mathematical concepts to use them effectively.
-
Master fundamental concepts: A strong grasp of fractions, decimals, and the relationships between them is essential.
-
Seek additional resources: Utilize online tutorials, educational websites, or textbooks for further learning and practice.
Conclusion
Converting the fraction 4/7 to a percentage is a fundamental mathematical skill with broad applicability. By mastering this conversion and understanding the underlying concepts, you'll gain a strong foundation for tackling more complex mathematical problems and real-world applications involving percentages. Remember that accuracy is crucial, and the method you choose will often depend on the level of precision required. Regular practice and a strong grasp of mathematical fundamentals are key to mastering these calculations. Through understanding and application, you can confidently navigate the world of percentages and utilize this skill in various contexts.
Latest Posts
Latest Posts
-
How Many Milliliters Are In 8 Fluid Ounces
May 09, 2025
-
How Many Feet Is 1 8th Mile
May 09, 2025
-
How Many Feet In 168 Inches
May 09, 2025
-
Kilometers Per Second To Meters Per Second
May 09, 2025
-
How Many Gallons In A Square Inch
May 09, 2025
Related Post
Thank you for visiting our website which covers about What Is The Percent Of 4/7 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.